Bagaimana Anda melacak jalur Pencarian Breadth-First, seperti dalam contoh berikut:
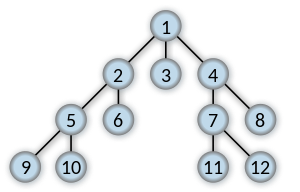
Jika mencari kunci 11, kembalikan daftar terpendek yang menghubungkan 1 hingga 11.
[1, 4, 7, 11]Bagaimana Anda melacak jalur Pencarian Breadth-First, seperti dalam contoh berikut:

Jika mencari kunci 11, kembalikan daftar terpendek yang menghubungkan 1 hingga 11.
[1, 4, 7, 11]Jawaban:
Anda harus melihat http://en.wikipedia.org/wiki/Breadth-first_search terlebih dahulu.
Di bawah ini adalah implementasi cepat, di mana saya menggunakan daftar daftar untuk mewakili antrian jalur.
# graph is in adjacent list representation
graph = {
'1': ['2', '3', '4'],
'2': ['5', '6'],
'5': ['9', '10'],
'4': ['7', '8'],
'7': ['11', '12']
}
def bfs(graph, start, end):
# maintain a queue of paths
queue = []
# push the first path into the queue
queue.append([start])
while queue:
# get the first path from the queue
path = queue.pop(0)
# get the last node from the path
node = path[-1]
# path found
if node == end:
return path
# enumerate all adjacent nodes, construct a new path and push it into the queue
for adjacent in graph.get(node, []):
new_path = list(path)
new_path.append(adjacent)
queue.append(new_path)
print bfs(graph, '1', '11')Pendekatan lain akan mempertahankan pemetaan dari setiap node ke induknya, dan saat memeriksa node yang berdekatan, catat induknya. Setelah pencarian selesai, cukup lacak balik sesuai pemetaan induk.
graph = {
'1': ['2', '3', '4'],
'2': ['5', '6'],
'5': ['9', '10'],
'4': ['7', '8'],
'7': ['11', '12']
}
def backtrace(parent, start, end):
path = [end]
while path[-1] != start:
path.append(parent[path[-1]])
path.reverse()
return path
def bfs(graph, start, end):
parent = {}
queue = []
queue.append(start)
while queue:
node = queue.pop(0)
if node == end:
return backtrace(parent, start, end)
for adjacent in graph.get(node, []):
if node not in queue :
parent[adjacent] = node # <<<<< record its parent
queue.append(adjacent)
print bfs(graph, '1', '11')Kode di atas didasarkan pada asumsi bahwa tidak ada siklus.
Saya sangat menyukai jawaban pertama qiao! Satu-satunya hal yang hilang di sini adalah menandai simpul sebagai telah dikunjungi.
Mengapa kita perlu melakukannya?
Mari kita bayangkan bahwa ada node lain nomor 13 yang terhubung dari node 11. Sekarang tujuan kita adalah menemukan node 13.
Setelah sedikit dijalankan, antrian akan terlihat seperti ini:
[[1, 2, 6], [1, 3, 10], [1, 4, 7], [1, 4, 8], [1, 2, 5, 9], [1, 2, 5, 10]]Perhatikan bahwa ada DUA jalur dengan simpul nomor 10 di akhir.
Artinya jalur dari node nomor 10 akan diperiksa dua kali. Dalam hal ini tidak terlihat terlalu buruk karena node nomor 10 tidak memiliki anak .. Tetapi bisa sangat buruk (bahkan di sini kita akan memeriksa node itu dua kali tanpa alasan ..)
Node nomor 13 tidak ada jalur tersebut sehingga program tidak akan kembali sebelum mencapai jalur kedua dengan node nomor 10 di akhir .. Dan kami akan memeriksanya kembali ..
Yang hilang hanyalah satu set untuk menandai node yang dikunjungi dan tidak untuk memeriksanya lagi ..
Ini adalah kode qiao setelah modifikasi:
graph = {
1: [2, 3, 4],
2: [5, 6],
3: [10],
4: [7, 8],
5: [9, 10],
7: [11, 12],
11: [13]
}
def bfs(graph_to_search, start, end):
queue = [[start]]
visited = set()
while queue:
# Gets the first path in the queue
path = queue.pop(0)
# Gets the last node in the path
vertex = path[-1]
# Checks if we got to the end
if vertex == end:
return path
# We check if the current node is already in the visited nodes set in order not to recheck it
elif vertex not in visited:
# enumerate all adjacent nodes, construct a new path and push it into the queue
for current_neighbour in graph_to_search.get(vertex, []):
new_path = list(path)
new_path.append(current_neighbour)
queue.append(new_path)
# Mark the vertex as visited
visited.add(vertex)
print bfs(graph, 1, 13)Output dari program ini adalah:
[1, 4, 7, 11, 13]Tanpa pemeriksaan ulang yang tidak perlu ..
collections.dequeuntuk queuesebagai list.pop (0) dikenakan O(n)gerakan memori. Juga, demi anak cucu, jika Anda ingin melakukan DFS cukup setel path = queue.pop()dalam hal ini variabel queuebenar-benar bertindak seperti a stack.
Kode yang sangat mudah. Anda terus menambahkan jalur setiap kali Anda menemukan sebuah node.
graph = {
'A': set(['B', 'C']),
'B': set(['A', 'D', 'E']),
'C': set(['A', 'F']),
'D': set(['B']),
'E': set(['B', 'F']),
'F': set(['C', 'E'])
}
def retunShortestPath(graph, start, end):
queue = [(start,[start])]
visited = set()
while queue:
vertex, path = queue.pop(0)
visited.add(vertex)
for node in graph[vertex]:
if node == end:
return path + [end]
else:
if node not in visited:
visited.add(node)
queue.append((node, path + [node]))Saya pikir saya akan mencoba kode ini untuk bersenang-senang:
graph = {
'1': ['2', '3', '4'],
'2': ['5', '6'],
'5': ['9', '10'],
'4': ['7', '8'],
'7': ['11', '12']
}
def bfs(graph, forefront, end):
# assumes no cycles
next_forefront = [(node, path + ',' + node) for i, path in forefront if i in graph for node in graph[i]]
for node,path in next_forefront:
if node==end:
return path
else:
return bfs(graph,next_forefront,end)
print bfs(graph,[('1','1')],'11')
# >>>
# 1, 4, 7, 11Jika Anda menginginkan siklus, Anda dapat menambahkan ini:
for i, j in for_front: # allow cycles, add this code
if i in graph:
del graph[i]Saya suka jawaban pertama @Qiao dan penambahan @ Or. Demi sedikit pemrosesan, saya ingin menambahkan jawaban Or.
Dalam jawaban @ Or melacak node yang dikunjungi sangat bagus. Kami juga dapat mengizinkan program untuk keluar lebih cepat dari saat ini. Di beberapa titik di loop for, current_neighbourharus menjadi end, dan begitu itu terjadi jalur terpendek ditemukan dan program dapat kembali.
Saya akan memodifikasi metode sebagai berikut, perhatikan loop for
graph = {
1: [2, 3, 4],
2: [5, 6],
3: [10],
4: [7, 8],
5: [9, 10],
7: [11, 12],
11: [13]
}
def bfs(graph_to_search, start, end):
queue = [[start]]
visited = set()
while queue:
# Gets the first path in the queue
path = queue.pop(0)
# Gets the last node in the path
vertex = path[-1]
# Checks if we got to the end
if vertex == end:
return path
# We check if the current node is already in the visited nodes set in order not to recheck it
elif vertex not in visited:
# enumerate all adjacent nodes, construct a new path and push it into the queue
for current_neighbour in graph_to_search.get(vertex, []):
new_path = list(path)
new_path.append(current_neighbour)
queue.append(new_path)
#No need to visit other neighbour. Return at once
if current_neighbour == end
return new_path;
# Mark the vertex as visited
visited.add(vertex)
print bfs(graph, 1, 13)Output dan yang lainnya akan sama. Namun, kode akan membutuhkan waktu lebih sedikit untuk diproses. Ini sangat berguna pada grafik yang lebih besar. Saya harap ini membantu seseorang di masa depan.